Fixed Media, Construction Method 

Point of departure: the converging series pi = 4(1 - 1/3 + 1/5 - 1/7 + 1/9 ...). 

Each convergence gets a time window of 5040 samples (twice the lowest common multiple of the numbers 1-10), in which ten square wave partials of frequencies 8¾n Hz and amplitude 2^d are set up, '8¾' deriving from the 5040 samples, 'n' being the partial number and 'd' the n-th digit in the convergence's decimal representation; e.g. for '3.141592654', the ten partials' amplitudes are 213, 211, 214, 211,215, 219 etc., thereafter rescaled by the sawtooth-spectral factor 2pi/n, where 'n' is still the partial number. The convergences make the digits stabilize from left to right to a value approaching pi, the resultant timbre moving from turbulence to constancy over 4 x 1019 x 5040 = 20.16 x 10^12 samples or ~14½ years. The installation can be pitch-shifted (by sample-dropping) and/or time-truncated. Here the six sound channels are transposed from 8¾ Hz to frequencies 9, 28, 50, 72, 96 and 123 times higher; the duration is a number of hours, during which the leftmost digits can be seen to gradually stabilize.

Fixed media 

13C2=Chronometrie

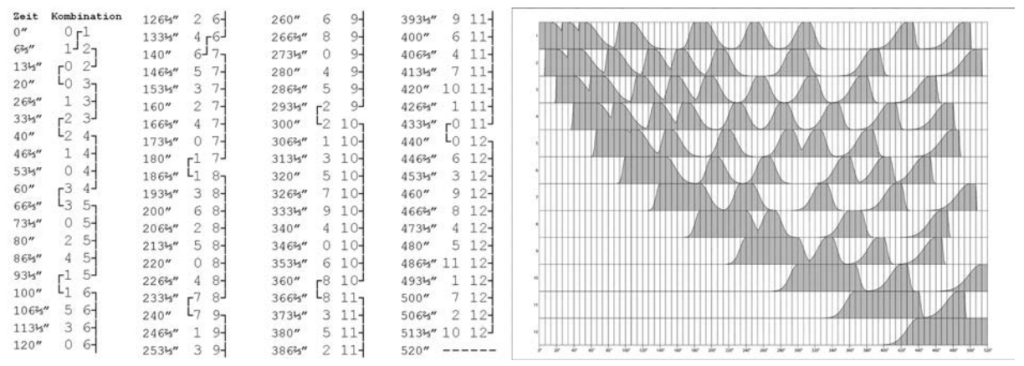
13C2=[♄] (pronunciation: "2 out of 13 chronometry") is the fifth in a series of twelve-channel electronic pieces created in 2016-2017, all of which share the fade map shown below, consisting of a fixed permutation of 78 of two combinations of 13 sound tracks. 

13C2=[♄] is a percussive piece in which twelve "instruments" in twelve sound tracks play a perpetuum  mobile in a total of 48 metres, each containing one to twelve pulses, nine per second. Each track begins in a metre with a number of pulses equal to the track number. Each metre (except the one with only one pulse) corresponds to a unique permutation of groups of two and/or three pulses, e.g. in the three 7-pulse metres 2+2+3, 2+3+2 and 3+2+2.   

The metres with a length of 12 pulses are twelve in number. At 6'13⅓" the metres begin to increase in length - track by track - until 7'20", where all twelve tracks contain synchronised 12-pulse metres of equal length but different lengths.    

 The "instruments" in question here are synthetic timbral interpolations between sine (track 1) and sawtooth (track 12) oscillations. Inspired by the work of J. M. Grey et al. (1978), the spectra become brighter in tone colour from track to track due to the addition of a rising formant-like structure ("spectral centroid") with an increase in attack time. The fundamental pitches of these spectra - rising chromatically from 110 to 208 Hz (A to G sharp) - correspond to the metric priority of the pulses calculated by me. 

Daan Vandewalle (piano) 

Çoǧluotobüsişletmesi is a thirty-minute piano piece, the composition of which was made possible by extensive research in (among other things) the fields of tonality and metricism. This is an account of this research. 

For example, it has been felt in various parts of the world and since antiquity that certain intervals - octave and fifth, for instance possess a greater degree of harmonicity than the others (e.g. the tritone).  Furthermore, in the more harmonic intervals, one of the two (termed the root) seems to carry greater weight than the other - the role of root is ascribed in e.g. the octave, fifth and major third to the lower note, but in the perfect fourth and minor sixth, the polarisation is found to be reversed. For several centuries now, various slightly divergent lists displaying the conjectured relative harmonicity of the twelve chromatic intervals have been appearing, in recent times with reference to their polarity as well; what has been lacking, however, is an explicit, consistent method bearing convincing results, capable of application to all kinds of intervals, including such e.g. with quartertone tuning (and this piece was to contain some). By means of a specifically developed formula yielding a complexity or indigestibility index for natural numbers (e.g. the values for the numbers one to nine indicate an increasing indigestibility in the order 1-2-4-3-8-6-9-5-7) it was found possible to evaluate a tonality coefficient for any desired interval, given its tuning as a ratio of whole numbers (e.g. the fifth, fourth and major third  were found to have tonalities of +0,273, -0,214 and +0.119, respectively, the plus and minus signs indicating the position of the root in the interval: Plus = lower note, minus = upper). Tempered intervals - dodecaphonic and microintervallic - were rationalised by searching in their immediate neighbourhood for the tuning with the highest tonality value (a test with the twelve tempered intervals in an octave produced tunings practically identical with just intonation), It was now possible to set up tonal fields of varying strength, in that in a given mode with a given tonic, the degree of desirability or preference accorded to each Pitch would be determined on the basis of its tonality, measured against the tonic. 

Similarly in the field of metricism it has been long felt that certain pulses in a given metre - the first and third in a four-beat-bar, for example - are stronger, more necessary for a characterisation of the metre than the others. Bymeans of a formula yielding an indispensibility index for each Pulse in any given metre (e.g. in a 6/8 bar the values for the eighthnotes were found to be 5-0-2-4-1-3, as against 5-0-3-1-4-2 for 3/4) it was possible to set upmetric fields of varying strength, in that in a given metre, the degree of desirability of attack on each of the Pulses could be determined via the indispensibility value -:- it was realised that the desirability of pitches was interrelated with that of the pulses which were to carry them - the combined desirability value was converted into reality by a system of priority and probability linked with random choice. 

Another field which was delved into was that of consonance (distinct from harmonicity!: this has to do with the Physical roughness certain intervals are observed to engender in the hearing mechanism). Based on the work of  acousticians and aural physiologists such as Plomp and Levelt, Zwicker and  Feldtkeller, Fletcher and Munson, a measure of consonance was developed, which worked for any interval, given the timbre of the instrument sounded. It was  found, for example, that in the lowest octave of the piano, a perfect fourth sounds rougher, more dissonant than a tritone, despite the former's greater harmonic content. 

The methods outlined here for the treatment of consonance, tonality and metre are so general as to be reemployable in totally different contexts. 

A word concerning material and organization: Çoǧluotobüsişletmesi  was composed for retuned piano, the same four notes in every octave being lowered by exactly one quartertone, On the Pitch (or 'microtemporal') level it is a modal piece, deriving entirely from twelve seven-note modal cycles, in which any two consecutive scale-degrees are always a half- to a wholetone apart (only three such modal cycles exist without microtonal retuning, one of these comprising the classical ecclesiastical cycle of seven modes). Patterns of regular beatsgrouped and divided (two 'orders' of metric organization) to form six different metres in each of fourteen tempiform the basis for the rhythmic ( 'macrotemporal') aspect of the piece. 

The most important general concept underlying this composition is the organisation of texture, defined in this context as being a result of the conformity between various parallel musical streams (in each of which no two events - notes or chords - ever overlap in time) of differing and varying consistency. Conformity here implies the degree or material similarity (of keys and/or metre) between streams, consistency the interaction of eight parameters: harmonic and metric cohesion (ranging down to atonality and ametricism), melodic and  (ranging  to pointillism and syncopation), chordal and tactile density (the quantity of pitches per chord, of chords per unit time), as well as dynamics (loudness) and articulation (connectedness). 

The pianist Daan Vandewalle is an internationally acclaimed performer of 20th and 21st century piano music. His repertoire consists of hundreds of pieces, integrales of most of the well-known composers of the 20th century such as Ives, Messiaen, Schonberg as well as numerous premieres that resulted from intense collaborations with composers of today. 

He has performed in a wide range of venues, from small clubs in the underground, experimental music scene to established venues such as the Prague Spring Festival, the Lincoln Centre en Carnegie Hall New York, Auditorio Nacional Madrid, the Mozarteum Salzburg, Theatre des Chatelets Paris, Konzerthaus Berlin and many others. 

He premiered dozens of new pieces e.g. Frederic Rzewski, Clarence Barlow, Maria De Alvear, Fred Frith and Gordon Mumma. He is one of the only pianists in the world who play the entire Opus Clavicembalisticum by Sorabji. Apart from his work as a soloist he forms a piano-duo with the legendary Australian pianist Geoffrey Douglas Madge, and duos with cellist Arne Deforce and singer Salome Kammer. 

Since 2001 he teaches piano at the conservatory of Ghent, University of Ghent College.

Photo credit: DaanVandewalle2 © Fred Debrock